Đề bài

Khảo sát sự biến thiên và vẽ đồ thị của các hàm số đã học theo sơ đồ trên

y=ax+b

y=a$x^2$+bx+c

Lời giải chi tiết

* Hàm số $y=ax+b$

Trường hợp a > 0

1. TXĐ: D=R$.$

2. Sự biến thiên.

$y\text{'}=a>0$. Vậy hàm số đồng biến trên toàn bộ R.

$$\begin{gathered} \underset{x\to +\infty }{\lim }y=+\infty \\ \underset{x\to -\infty }{\lim }y=-\infty \end{gathered}$$

Bảng biến thiên

3. Vẽ đồ thị

Trường hợp a<0

1. TXĐ: D=R$.$

2. Sự biến thiên.

$y\text{'}=a<0$. Vậy hàm số đồng biến trên toàn bộ R.

$$\begin{gathered} \underset{x\to +\infty }{\lim }y=-\infty \\ \underset{x\to -\infty }{\lim }y=+\infty \end{gathered}$$

Bảng biến thiên

Vẽ đồ thị

* Hàm số $y=a{x}^2+bx+c$

Trường hợp $a>0$

1. TXĐ: D=R

2. Sự biến thiên.

$y\text{'}=2ax+b.$

$y\text{'}=0\Rightarrow x=\frac{-b}{2a}$

$$\begin{gathered} \underset{x\to +\infty }{\lim }y=+\infty \\ \underset{x\to -\infty }{\lim }y=+\infty \end{gathered}$$

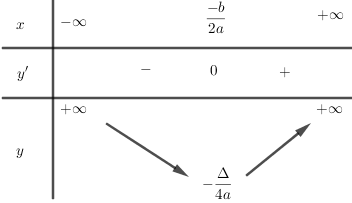
Bảng biến thiên

Hàm số nghịch biến trên khoảng $\left(-\infty , \frac{-b}{2a}\right)$.

Hàm số đồng biến trên khoảng $\left(\frac{-b}{2a}, +\infty \right)$.

Hàm số đạt cực tiểu bằng $\frac{-∆}{4a}$ tại $x=\frac{-b}{2a}$

Vẽ đồ thị

Trường hợp $a<0$

1. TXĐ: D=R$.$

2. Sự biến thiên.

$y\text{'}=2ax+b$

Cho $y\text{'}=0\Rightarrow x=\frac{-b}{2a}$

$$\begin{gathered} \underset{x\to +\infty }{\lim }y=-\infty \\ \underset{x\to -\infty }{\lim }y=-\infty \end{gathered}$$

Bảng biến thiên

Hàm số đồng biến trên khoảng $\left(-\infty , \frac{-b}{2a}\right)$.

Hàm số nghịch biến trên khoảng $\left(\frac{-b}{2a}, +\infty \right)$.

Hàm số đạt cực đại bằng $\frac{-∆}{4a}$ tại $x=\frac{-b}{2a}$

Vẽ đồ thị