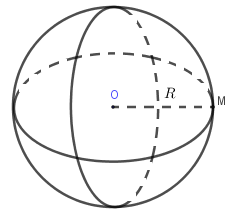
1. Định nghĩa:

Tập hợp các điểm trong không gian cách điểm $O$ cố định một khoảng không đổi $r\left(r>0\right)$ được gọi là

một mặt cầu tâm $O$ bán kính $r$ .

Kí hiệu: $S\left(O; R\right)=\left\{M\overline{) OM=R}\right\}$

* Cho mặt cầu $S\left(O; r\right)$ và điểm $A$ trong không gian.

- Nếu $OA=r$ thì điểm $A$ nằm trên mặt cầu

- Nếu $OA<r$ thì điểm $A$ nằm trong mặt cầu.

- Nếu $OA>r$ thì điểm $A$ nằm ngoài mặt cầu.

2. Tính chất:

Nếu điểm $A$ ngoài mặt cầu $S\left(O; r\right)$ thì:

- Qua $A$ có vô số tiếp tuyến với mặt cầu.

- Độ dài các đoạn thẳng nối $A$ với các tiếp điểm đều bằng nhau.

- Tập hợp các tiếp điểm là một đường tròn nằm trên mặt cầu.

3. Giao của mặt cầu với mặt phẳng

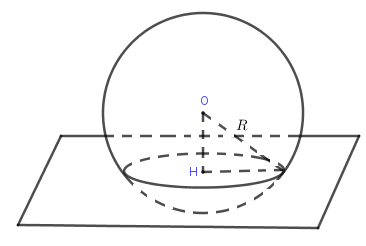
Cho mặt cầu $\left(S\right)$ tâm $O$ , bán kính $R$ và mặt phẳng $\left(P\right)$, gọi $H$ là hình chiếu của $O$ trên $\left(P\right)$ .

+ Nếu $OH<R$ thì $\left(S\right)$ cắt $\left(P\right)$ theo đường tròn tâm $H$ và bán kính $r=\sqrt{R^2-O{H}^2}$ .

+ Nếu $OH=R$ thì $\left(S\right)$ tiếp xúc $\left(P\right)$ tại tiếp điểm $H$.

+ Nếu $OH>R$ thì $\left(S\right)$ và $\left(P\right)$ không có điểm chung.

Đặc biệt: Nếu $OH=0 \left(O\equiv H\right)$ thì đường tròn giao tuyến của $\left(P\right)$và $\left(S\right)$ được gọi là đường tròn lớn, $\left(P\right)$ được

gọi là mặt phẳng kính.

4. Giao của mặt cầu với đường thẳng.

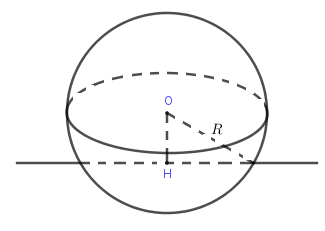
Cho mặt cầu $\left(S\right)$ tâm $O$ , bán kính $R$ và đường thẳng $d$, gọi $H$ là hình chiếu của $O$ trên $d$.

+ Nếu $OH<R$ thì $\left(S\right)$ cắt $d$ tại 2 điểm phân biệt.

+ Nếu $OH=R$ thì $\left(S\right)$ cắt $d$ tại một điểm duy nhất $H$ ($d$ là tiếp tuyến với mặt cầu, $H$ là tiếp điểm)

+ Nếu $OH>R$ thì $\left(S\right)$ và $d$ không có điểm chung.

5. Tiếp tuyến với mặt cầu (Đọc thêm)

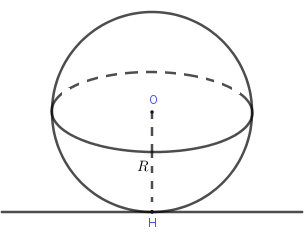
- Qua một điểm nằm trong mặt cầu không vẽ được tiếp tuyến nào với mặt cầu.

- Qua một điểm nằm trên mặt cầu vẽ được vô số tiếp tuyến với mặt cầu tại điểm đó. Tập hợp các tiếp tuyến

chính là mặt phẳng tiếp diện của mặt cầu.

- Qua một điểm nằm ngoài mặt cầu vẽ được vô số tiếp tuyến với mặt cầu. Tập hợp các tiếp điểm với mặt cầu

là đường tròn nằm trên mặt cầu.

6. Công thức diện tích mặt cầu và thể tích hình cầu