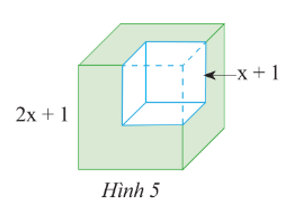
Vận dụng 4 trang 22 Toán 8 tập 1

Vận dụng 4 trang 22 Toán 8 Tập 1: Từ một khối lập phương có cạnh bằng 2x + 1, ta cắt bỏ một khối lập phương có cạnh bằng x + 1 (xem Hình 5). Tính thể tích phần còn lại, viết kết quả dưới dạng đa thức. <div class="ads_ads ads_1"><ins class="adsbygoogle" data-ad-client="ca-pub-2373417360267295" data-ad-slot="9405778504" data-ad-format="auto" data-full-width-responsive="true" data-adsbygoogle-status="done" data-ad-status="unfilled"> <div id="aswift_1_host" tabindex="0" title="Advertisement" aria-label="Advertisement"></div> </ins></div> <img src="https://vietjack.com/toan-8-ct/images/van-dung-4-trang-22-toan-8-tap-1.PNG" alt="Vận dụng 4 trang 22 Toán 8 Tập 1 Chân trời sáng tạo | Giải Toán 8" /> Lời giải: Thể tích của khối lập phương có cạnh bằng 2x + 1 là: (2x + 1)3. Thể tích của khối lập phương có cạnh bằng x + 1 là: (x + 1)3. Cách 1: Thể tích phần còn lại là: (2x + 1)3 – (x + 1)3 = (2x)3 + 3.(2x)2.1 + 3.2x.12 + 13 – (x3 + 3.x2.1 + 3.x.12 + 13) = 8x3 + 12x2 + 6x + 1 – x3 – 3x2 – 3x – 1 = (8x3 –  x3) + (12x2 – 3x2) + (6x – 3x) + (1 – 1) = 7x3 + 9x2 + 3x. Cách 1: Thể tích phần còn lại là: (2x + 1)3 – (x + 1)3 = [(2x + 1) – (x + 1)].[(2x + 1)2 + (2x + 1).(x + 1) + (x + 1)2] = [2x + 1 – x – 1].[(2x)2 + 2.2x.1 + 12 + (2x.x + 2x.x + 1.x + 1.1) + x2 + 2.x.1 + 12] = x.[4x2 + 4x + 1 + 2x2 + 3x + 1 + x2 + 2x + 1] = x.[(4x2 + 2x2 + x2) + (4x + 3x + 2x) + (1 + 1 + 1)] = x.[7x2 + 9x + 3] = 7x3 + 9x2 + 3x.

Xem lời giải bài tập khác cùng bài