Bài 3: Tính Chất Đường Phân Giác Của Tam Giác
Hướng dẫn giải Bài 22 (Trang 68 SGK Toán Hình học 8, Tập 2)
<p>Đề bài<br />Đố: Hình 27 cho biết có 6 góc bằng nhau:<br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>⁢</mo><mover accent="true"><msub><mi>O</mi><mn>1</mn></msub><mo>^</mo></mover><mo>=</mo><mover accent="true"><msub><mi>O</mi><mn>2</mn></msub><mo>^</mo></mover><mo>=</mo><mover accent="true"><msub><mi>O</mi><mn>3</mn></msub><mo>^</mo></mover><mo>=</mo><mover accent="true"><msub><mi>O</mi><mn>4</mn></msub><mo>^</mo></mover><mo>=</mo><mover accent="true"><msub><mi>O</mi><mn>5</mn></msub><mo>^</mo></mover><mo>=</mo><mover accent="true"><msub><mi>O</mi><mn>6</mn></msub><mo>^</mo></mover><mo>⁢</mo><mo>.</mo></math><br />Kích thước các đoạn thẳng đã được ghi trên hình. Hãy thiết lập những tỉ lệ thức từ' kích thước đã cho.</p>
<p><img src="https://img.loigiaihay.com/picture/2018/0718/b22-trang-68-sgk-toan-8-t2-c2.jpg" /></p>
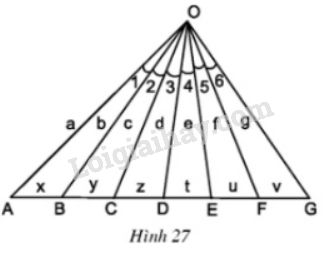
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p>OB là đường phân giác trong của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>C</mi><mo>⇒</mo><mfrac><mi>x</mi><mi>a</mi></mfrac><mo>=</mo><mfrac><mi>y</mi><mi>c</mi></mfrac></mstyle></math><br />OC là đường phân giác trong của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>D</mi><mo>⇒</mo><mfrac><mi>y</mi><mi>b</mi></mfrac><mo>=</mo><mfrac><mi>z</mi><mi>d</mi></mfrac></mstyle></math><br />OD là đường phân giác trong của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>C</mi><mo>⁢</mo><mi>E</mi><mo>⇒</mo><mfrac><mi>z</mi><mi>c</mi></mfrac><mo>=</mo><mfrac><mi>t</mi><mi>e</mi></mfrac></mstyle></math><br />OE là đường phân giác trong của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>D</mi><mo>⁢</mo><mi>F</mi><mo>⇒</mo><mfrac><mi>t</mi><mi>d</mi></mfrac><mo>=</mo><mfrac><mi>u</mi><mi>f</mi></mfrac></mstyle></math><br />OF là đường phân giác trong của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⁢</mo><mi>G</mi><mo>⇒</mo><mfrac><mi>u</mi><mi>e</mi></mfrac><mo>=</mo><mfrac><mi>v</mi><mi>g</mi></mfrac></mstyle></math><br />OC là đường phân giác của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⇒</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><mi>O</mi><mo>⁢</mo><mi>A</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>C</mi><mo>⁢</mo><mi>E</mi></mrow><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow></mfrac></mstyle></math> hay <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>x</mi><mo>+</mo><mi>y</mi></mrow><mi>a</mi></mfrac><mo>=</mo><mfrac><mrow><mi>z</mi><mo>+</mo><mi>t</mi></mrow><mi>e</mi></mfrac></mstyle></math><br />OE là đường phân giác của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>C</mi><mo>⁢</mo><mi>G</mi><mo>⇒</mo><mfrac><mrow><mi>z</mi><mo>+</mo><mi>t</mi></mrow><mi>c</mi></mfrac><mo>=</mo><mfrac><mrow><mi>u</mi><mo>+</mo><mi>v</mi></mrow><mi>g</mi></mfrac></mstyle></math><br />OD làđường phân giác của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>G</mi><mo>⇒</mo></mstyle></math> <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>x</mi><mo>+</mo><mi>y</mi><mo>+</mo><mi>z</mi></mrow><mi>a</mi></mfrac><mo>=</mo><mfrac><mrow><mi>t</mi><mo>+</mo><mi>u</mi><mo>+</mo><mi>v</mi></mrow><mi>g</mi></mfrac></mstyle></math><br />OD là đường phân giác của <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>F</mi><mo>⇒</mo><mfrac><mrow><mi>y</mi><mo>+</mo><mi>z</mi></mrow><mi>b</mi></mfrac><mo>=</mo><mfrac><mrow><mi>t</mi><mo>+</mo><mi>u</mi></mrow><mi>f</mi></mfrac></mstyle></math></p>
Xem lời giải bài tập khác cùng bài
Hướng dẫn giải Bài 16 (Trang 67 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 17 (Trang 67 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 18 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 19 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 20 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 21 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải