Bài 3: Tính Chất Đường Phân Giác Của Tam Giác
Hướng dẫn giải Bài 20 (Trang 68 SGK Toán Hình học 8, Tập 2)
<p><strong class="content_question">Đề bài</strong></p>
<p>Cho hình thang <span id="MathJax-Element-1-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi><mi>D</mi><mspace width="thickmathspace" /><mo stretchy="false">(</mo><mi>A</mi><mi>B</mi><mrow class="MJX-TeXAtom-ORD"><mo>/</mo></mrow><mrow class="MJX-TeXAtom-ORD"><mo>/</mo></mrow><mi>C</mi><mi>D</mi><mo stretchy="false">)</mo></math>"><span id="MJXc-Node-1" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-2" class="mjx-mrow"><span id="MJXc-Node-3" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-4" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span><span id="MJXc-Node-5" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span><span id="MJXc-Node-6" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">D</span></span><span id="MJXc-Node-7" class="mjx-mspace"></span><span id="MJXc-Node-8" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">(</span></span><span id="MJXc-Node-9" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-10" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span><span id="MJXc-Node-11" class="mjx-texatom"><span id="MJXc-Node-12" class="mjx-mrow"><span id="MJXc-Node-13" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">/</span></span></span></span><span id="MJXc-Node-14" class="mjx-texatom"><span id="MJXc-Node-15" class="mjx-mrow"><span id="MJXc-Node-16" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">/</span></span></span></span><span id="MJXc-Node-17" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span><span id="MJXc-Node-18" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">D</span></span><span id="MJXc-Node-19" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">)</span></span></span></span></span>. Hai đường chéo <span id="MathJax-Element-2-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>C</mi></math>"><span id="MJXc-Node-20" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-21" class="mjx-mrow"><span id="MJXc-Node-22" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-23" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span></span></span></span> và <span id="MathJax-Element-3-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>B</mi><mi>D</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>B</mi><mi>D</mi></math></span></span> cắt nhau tại <span id="MathJax-Element-4-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi></math>"><span id="MJXc-Node-28" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-29" class="mjx-mrow"><span id="MJXc-Node-30" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">O</span></span></span></span></span>. Đường thẳng <span id="MathJax-Element-5-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>a</mi></math>"><span id="MJXc-Node-31" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-32" class="mjx-mrow"><span id="MJXc-Node-33" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">a</span></span></span></span></span> qua <span id="MathJax-Element-6-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi></math>"><span id="MJXc-Node-34" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-35" class="mjx-mrow"><span id="MJXc-Node-36" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">O</span></span></span></span></span> và song song với đáy của hình thang cắt các cạnh <span id="MathJax-Element-7-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>D</mi><mo>,</mo><mi>B</mi><mi>C</mi></math>"><span id="MJXc-Node-37" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-38" class="mjx-mrow"><span id="MJXc-Node-39" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-40" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">D</span></span><span id="MJXc-Node-41" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">,</span></span><span id="MJXc-Node-42" class="mjx-mi MJXc-space1"><span class="mjx-char MJXc-TeX-math-I">B</span></span><span id="MJXc-Node-43" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span></span></span></span> theo thứ tự <span id="MathJax-Element-8-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>E</mi></math>"><span id="MJXc-Node-44" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-45" class="mjx-mrow"><span id="MJXc-Node-46" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">E</span></span></span></span></span> và <span id="MathJax-Element-9-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>F</mi></math>"><span id="MJXc-Node-47" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-48" class="mjx-mrow"><span id="MJXc-Node-49" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">F</span></span></span></span></span> (h26)</p>
<p>Chứng minh rằng OE=OF.</p>
<p><img src="https://img.loigiaihay.com/picture/2018/0718/b20-trang-68-sgk-toan-8-t2-c2.jpg" /></p>
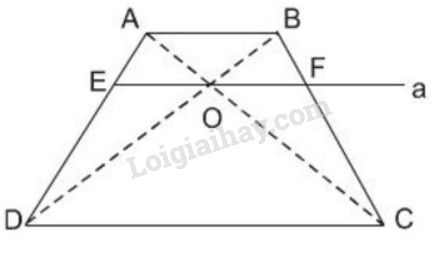
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p><span class="content_detail"><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>D</mi><mo>⁢</mo><mi>C</mi></mstyle></math> có OE//DC (gt) nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mrow><mi>D</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi></mrow><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac></mstyle></math> (1) (hệ quả của định lí TaLet trong tam giác)</span></p>
<p><span class="content_detail"> <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>D</mi><mo>⁢</mo><mi>C</mi></mstyle></math> có OF//DC (gt) nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>O</mi><mo>⁢</mo><mi>F</mi></mrow><mrow><mi>D</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow><mrow><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac></mstyle></math> (2) (hệ quả của định lí TaLet trong tam giác) </span></p>
<p><span class="content_detail"><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">△</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>C</mi></mstyle></math> có OF//AB (gt) nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi></mrow><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow><mrow><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac></mstyle></math> (3) (hệ quả của định lí TaLet trong tam giác) </span></p>
<p><span class="content_detail">Từ (1), (2), (3) suy ra <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mrow><mi>D</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>O</mi><mo>⁢</mo><mi>F</mi></mrow><mrow><mi>D</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac></mstyle></math> nên OE=OF.<br /></span></p>
<p> </p>
Xem lời giải bài tập khác cùng bài
Hướng dẫn giải Bài 16 (Trang 67 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 17 (Trang 67 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 18 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 19 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 21 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải
Hướng dẫn giải Bài 22 (Trang 68 SGK Toán Hình học 8, Tập 2)
Xem lời giải