Bài 1: Hệ tọa độ trong không gian
Lý thuyết Hệ tọa độ trong không gian
<p><strong>1. Hệ tọa độ trong không gian</strong></p>
<p>Trong không gian cho ba trục tọa độ chung gốc <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi></math>, đôi một vuông góc với nhau <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>x</mi><mo>'</mo><mi>O</mi><mi>x</mi><mo>;</mo><mo> </mo><mi>y</mi><mo>'</mo><mi>O</mi><mi>y</mi><mo>;</mo><mo> </mo><mi>z</mi><mo>'</mo><mi>O</mi><mi>z</mi></math>. Hệ ba</p>
<p>trục tọa độ như vậy được gọi là hệ trục tọa độ Đề-các vuông góc <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>x</mi><mi>y</mi><mi>z</mi></math>; <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi></math> là gốc tọa tọa độ. Giả sử <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>i</mi><mo>→</mo></mover><mo>,</mo><mo> </mo><mover><mi>j</mi><mo>→</mo></mover><mo>,</mo><mo> </mo><mover><mi>k</mi><mo>→</mo></mover></math> </p>
<p>lần lượt là các vectơ đơn vị trên các trục <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>x</mi><mo>'</mo><mi>O</mi><mi>x</mi><mo>,</mo><mo> </mo><mi>y</mi><mo>'</mo><mi>O</mi><mi>y</mi><mo>,</mo><mo> </mo><mi>z</mi><mo>'</mo><mi>O</mi><mi>z</mi></math> (h. 52)</p>
<p><img src="https://img.loigiaihay.com/picture/2021/1027/hetoado.png" width="302" height="278" /></p>
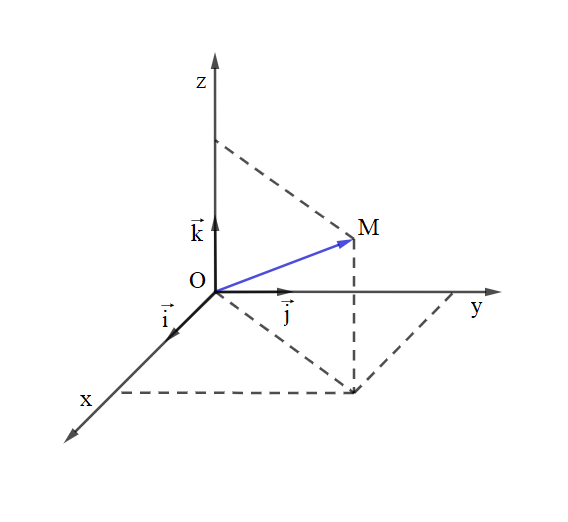
<p>Với điểm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>M</mi></math> thuộc không gian <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>x</mi><mi>y</mi><mi>z</mi></math> thì tồn tại duy nhất bộ số <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>x</mi><mo>;</mo><mo> </mo><mi>y</mi><mo>;</mo><mo> </mo><mi>z</mi></mrow></mfenced></math> để <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>O</mi><mi>M</mi></mrow><mo>→</mo></mover><mo>=</mo><mi>x</mi><mo>.</mo><mover><mi>i</mi><mo>→</mo></mover><mo>+</mo><mi>y</mi><mo>.</mo><mover><mi>j</mi><mo>→</mo></mover><mo>+</mo><mi>z</mi><mo>.</mo><mover><mi>k</mi><mo>→</mo></mover><mo>,</mo></math> bộ <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>x</mi><mo>;</mo></mrow></mfenced></math></p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mo> </mo><mi>y</mi><mo>;</mo><mo><br /></mo><mi>z</mi></mrow></mfenced></math> được gọi là tọa độ của điểm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>M</mi><mfenced><mrow><mi>x</mi><mo>;</mo><mo> </mo><mi>y</mi><mo>;</mo><mi>z</mi></mrow></mfenced></math>.</p>
<p>Trong không gian Oxyz cho vectơ <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover></math>, khi đó <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>=</mo><msub><mi>a</mi><mn>1</mn></msub><mover><mi>i</mi><mo>→</mo></mover><mo>+</mo><msub><mi>a</mi><mn>2</mn></msub><mover><mi>j</mi><mo>→</mo></mover><mo>+</mo><msub><mi>a</mi><mn>3</mn></msub><mover><mi>k</mi><mo>→</mo></mover></math></p>
<p>Ta viết <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>;</mo><msub><mi>a</mi><mn>2</mn></msub><mo>;</mo><msub><mi>a</mi><mn>3</mn></msub></mrow></mfenced></math> và nói <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover></math> có tọa độ <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>;</mo><msub><mi>a</mi><mn>2</mn></msub><mo>;</mo><msub><mi>a</mi><mn>3</mn></msub></mrow></mfenced></math> .</p>
<p><strong>2. Biểu thức tọa độ của các phép toán vectơ</strong></p>
<p>Giả sử <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>=</mo><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>;</mo><msub><mi>a</mi><mn>2</mn></msub><mo>;</mo><msub><mi>a</mi><mn>3</mn></msub></mrow></mfenced></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>b</mi><mo>→</mo></mover><mo>=</mo><mfenced><mrow><msub><mi>b</mi><mn>1</mn></msub><mo>;</mo><msub><mi>b</mi><mn>2</mn></msub><mo>;</mo><msub><mi>b</mi><mn>3</mn></msub></mrow></mfenced></math> thì:</p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>+</mo><mover><mi>b</mi><mo>→</mo></mover><mo>=</mo><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>+</mo><msub><mi>b</mi><mn>1</mn></msub><mo>;</mo><mo> </mo><msub><mi>a</mi><mn>2</mn></msub><mo>+</mo><msub><mi>b</mi><mn>2</mn></msub><mo>;</mo><mo> </mo><msub><mi>a</mi><mn>3</mn></msub><mo>+</mo><msub><mi>b</mi><mn>3</mn></msub></mrow></mfenced><mo>.</mo></math><span id="MathJax-Element-28-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo>=</mo><mo stretchy="false">(</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>1</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>2</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>3</mn></msub></mrow><mo stretchy="false">)</mo><mo>.</mo></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo stretchy="false"></mo><mo><br /></mo></math></span></span></p>
<p><span class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo>=</mo><mo stretchy="false">(</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>1</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>2</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>3</mn></msub></mrow><mo stretchy="false">)</mo><mo>.</mo></math>"><math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>-</mo><mover><mi>b</mi><mo>→</mo></mover><mo>=</mo><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>-</mo><msub><mi>b</mi><mn>1</mn></msub><mo>;</mo><mo> </mo><msub><mi>a</mi><mn>2</mn></msub><mo>-</mo><msub><mi>b</mi><mn>2</mn></msub><mo>;</mo><mo> </mo><msub><mi>a</mi><mn>3</mn></msub><mo>-</mo><msub><mi>b</mi><mn>3</mn></msub><mo> </mo></mrow></mfenced></math>.</span></p>
<p><span class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo>=</mo><mo stretchy="false">(</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>1</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>2</mn></msub></mrow><mo>;</mo><mi>k</mi><mrow class="MJX-TeXAtom-ORD"><msub><mi>a</mi><mn>3</mn></msub></mrow><mo stretchy="false">)</mo><mo>.</mo></math>"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>k</mi><mo>.</mo><mo> </mo><mover><mi>a</mi><mo>→</mo></mover><mo>=</mo><mfenced><mrow><mi>k</mi><msub><mi>a</mi><mn>1</mn></msub><mo>;</mo><mo> </mo><mi>k</mi><msub><mi>a</mi><mn>2</mn></msub><mo>;</mo><mo> </mo><mi>k</mi><msub><mi>a</mi><mn>3</mn></msub></mrow></mfenced><mo>.</mo></math></span></p>
<p><strong>3. Tích vô hướng</strong></p>
<p>Cho và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mfenced><mrow><msub><mi>a</mi><mn>1</mn></msub><mo>;</mo><msub><mi>a</mi><mn>2</mn></msub><mo>;</mo><msub><mi>a</mi><mn>3</mn></msub></mrow></mfenced></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>b</mi><mo>→</mo></mover><mfenced><mrow><msub><mi>b</mi><mn>1</mn></msub><mo>;</mo><msub><mi>b</mi><mn>2</mn></msub><mo>;</mo><msub><mi>b</mi><mn>3</mn></msub></mrow></mfenced></math> thì tích vô hướng<math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>.</mo><mover><mi>b</mi><mo>→</mo></mover><mo>=</mo><msub><mi>a</mi><mn>1</mn></msub><mo>.</mo><msub><mi>b</mi><mn>1</mn></msub><mo>+</mo><mo> </mo><msub><mi>a</mi><mn>2</mn></msub><mo>.</mo><msub><mi>b</mi><mn>2</mn></msub><mo>+</mo><mo> </mo><msub><mi>a</mi><mn>3</mn></msub><mo>.</mo><msub><mi>b</mi><mn>3</mn></msub></math></p>
<p>Ta có: <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced open="|" close="|"><mover><mi>a</mi><mo>→</mo></mover></mfenced><mo>=</mo><msqrt><msubsup><mi>a</mi><mn>1</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>a</mi><mn>2</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>a</mi><mn>3</mn><mn>2</mn></msubsup></msqrt><mo>.</mo></math></p>
<p>Đặt <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>φ</mi><mo>=</mo><mfenced><mover><mrow><mover><mi>a</mi><mo>→</mo></mover><mo>,</mo><mover><mi>b</mi><mo>→</mo></mover></mrow><mo>^</mo></mover></mfenced><mo>,</mo><mo> </mo><mn>0</mn><mo>⩽</mo><mi>φ</mi><mo>⩽</mo><mn>180</mn><mo>°</mo></math> thì </p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>cos</mi><mi>φ</mi><mo>=</mo><mfrac><mrow><msub><mi>a</mi><mn>1</mn></msub><msub><mi>b</mi><mn>1</mn></msub><mo>+</mo><msub><mi>a</mi><mn>2</mn></msub><msub><mi>b</mi><mn>2</mn></msub><mo>+</mo><msub><mi>a</mi><mn>3</mn></msub><msub><mi>b</mi><mn>3</mn></msub></mrow><mrow><msqrt><msubsup><mi>a</mi><mn>1</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>a</mi><mn>2</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>a</mi><mn>3</mn><mn>2</mn></msubsup></msqrt><msqrt><msubsup><mi>b</mi><mn>1</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>b</mi><mn>2</mn><mn>2</mn></msubsup><mo>+</mo><msubsup><mi>b</mi><mn>3</mn><mn>2</mn></msubsup></msqrt></mrow></mfrac></math>(với <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mi>a</mi><mo>→</mo></mover><mo>≠</mo><mover><mn>0</mn><mo>→</mo></mover><mo>,</mo><mo> </mo><mover><mi>b</mi><mo>→</mo></mover><mo> </mo><mo>≠</mo><mover><mn>0</mn><mo>→</mo></mover></math>)<br /><strong>4. Phương trình mặt cầu</strong></p>
<p>Trong không gian <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>x</mi><mi>y</mi><mi>z</mi></math>, mặt cầu <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>S</mi></mfenced></math> tâm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mfenced><mrow><mi>a</mi><mo>;</mo><mo> </mo><mi>b</mi><mo>;</mo><mo> </mo><mi>c</mi></mrow></mfenced></math> bán kính <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>R</mi></math> có phương trình chính tắc </p>
<p> <math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mfenced><mrow><mi>x</mi><mo>-</mo><mi>a</mi></mrow></mfenced><mn>2</mn></msup><mo> </mo><mo>+</mo><mo> </mo><msup><mfenced><mrow><mi>y</mi><mo>-</mo><mi>b</mi></mrow></mfenced><mn>2</mn></msup><mo> </mo><mo>+</mo><mo> </mo><msup><mfenced><mrow><mi>z</mi><mo>-</mo><mi>c</mi></mrow></mfenced><mn>2</mn></msup><mo> </mo><mo>=</mo><mo> </mo><msup><mi>R</mi><mn>2</mn></msup></math></p>
<p>Mặt cầu có phương trình tổng quát </p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mi>x</mi><mn>2</mn></msup><mo>+</mo><msup><mi>y</mi><mn>2</mn></msup><mo>+</mo><msup><mi>z</mi><mn>2</mn></msup><mo>+</mo><mn>2</mn><mi>a</mi><mi>x</mi><mo>+</mo><mn>2</mn><mi>b</mi><mi>y</mi><mo>+</mo><mn>2</mn><mi>c</mi><mi>z</mi><mo>+</mo><mi>d</mi><mo>=</mo><mn>0</mn></math></p>
<p> có tâm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mfenced><mrow><mo>-</mo><mi>a</mi><mo>;</mo><mo>-</mo><mi>b</mi><mo>;</mo><mo>-</mo><mi>c</mi></mrow></mfenced></math> và bán kính <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>R</mi><mo>=</mo><msqrt><msup><mi>a</mi><mn>2</mn></msup><mo>+</mo><msup><mi>b</mi><mn>2</mn></msup><mo>+</mo><msup><mi>c</mi><mn>2</mn></msup><mo>-</mo><mi>d</mi></msqrt></math></p>
Xem lời giải bài tập khác cùng bài
Hướng dẫn giải Hoạt động 1 (Trang 63 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Hoạt động 2 (Trang 64 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Hoạt động 3 (Trang 66 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Hoạt động 4 (Trang 67 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 1 (Trang 68 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 2 (Trang 68 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 3 (Trang 68 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 4 (Trang 68 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 5 (Trang 68 SGK Toán Hình học 12)
Xem lời giải
Hướng dẫn giải Bài 6 (Trang 68 SGK Toán Hình học 12)
Xem lời giải