Ôn tập chương 2
Hướng dẫn giải Bài 43 (Trang 133 SGK Toán Hình học 8, Tập 1)
<p><strong class="content_question">Đề bài</strong></p>
<p>Cho hình vuông <span id="MathJax-Element-1-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi><mi>D</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi><mi>D</mi></math></span></span> có tâm đối xứng <span id="MathJax-Element-2-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi></math>"><span id="MJXc-Node-7" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-8" class="mjx-mrow"><span id="MJXc-Node-9" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">O</span></span></span></span></span>, cạnh <span id="MathJax-Element-3-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>a</mi><mo>.</mo></math>"><span id="MJXc-Node-10" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-11" class="mjx-mrow"><span id="MJXc-Node-12" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">a</span></span></span></span><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>.</mo></math></span></span> Một góc vuông <span id="MathJax-Element-4-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>x</mi><mi>O</mi><mi>y</mi></math>"><span id="MJXc-Node-14" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-15" class="mjx-mrow"><span id="MJXc-Node-16" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">x</span></span><span id="MJXc-Node-17" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">Oy</span></span></span></span></span> có tia <span id="MathJax-Element-5-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>x</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>x</mi></math></span></span> cắt cạnh <span id="MathJax-Element-6-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi></math></span></span> tại <span id="MathJax-Element-7-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>E</mi></math>"><span id="MJXc-Node-27" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-28" class="mjx-mrow"><span id="MJXc-Node-29" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">E</span></span></span></span></span>, tia <span id="MathJax-Element-8-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>y</mi></math>"><span id="MJXc-Node-30" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-31" class="mjx-mrow"><span id="MJXc-Node-32" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">O</span></span><span id="MJXc-Node-33" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">y</span></span></span></span></span> cắt cạnh <span id="MathJax-Element-9-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>B</mi><mi>C</mi></math>"><span id="MJXc-Node-34" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-35" class="mjx-mrow"><span id="MJXc-Node-36" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span></span></span><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>C</mi></math></span></span> tại <span id="MathJax-Element-10-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>F</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>F</mi></math></span></span> (h.<span id="MathJax-Element-11-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mn>161</mn></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mn>161</mn></math></span></span>)</p>
<p><img src="https://img.loigiaihay.com/picture/2018/0716/b43a-trang-133-sgk-toan-8-t-1-c2.jpg" /></p>
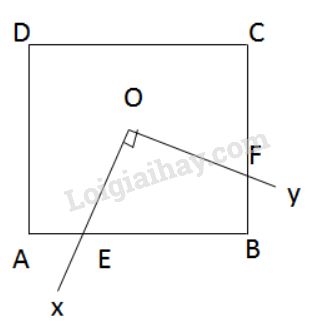
<p>Tính diện tích tứ giác <span id="MathJax-Element-12-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: 400; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: 0px; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; color: #000000; font-family: OpenSans, Tahoma, Helvetica, sans-serif; font-variant-ligatures: normal; font-variant-caps: normal; orphans: 2; widows: 2; -webkit-text-stroke-width: 0px; text-decoration-thickness: initial; text-decoration-style: initial; text-decoration-color: initial; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>O</mi><mi>E</mi><mi>B</mi><mi>F</mi><mo>.</mo></math>"><span id="MJXc-Node-44" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-45" class="mjx-mrow"><span id="MJXc-Node-46" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">O</span></span><span id="MJXc-Node-47" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">E</span></span><span id="MJXc-Node-48" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span><span id="MJXc-Node-49" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">F</span></span><span id="MJXc-Node-50" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">.</span></span></span></span></span><br /><strong class="content_detail">Lời giải chi tiết</strong></p>
<p><img src="https://img.loigiaihay.com/picture/2018/0716/b43b-trang-133-sgk-toan-8-t-1-c2.jpg" /></p>
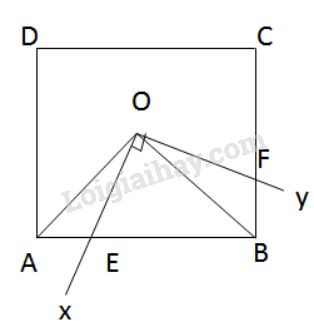
<p>Nối OA, OB.<br />Do ABCD là hình vuông nên O là trung điểm của AC, BD và <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi></mrow><mo>^</mo></mover><mo>=</mo><msup><mn>90</mn><mo>∘</mo></msup></mstyle></math><br />Ta có: <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover><mo>+</mo><mover accent="true"><mrow><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover><mo>=</mo><mover accent="true"><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi></mrow><mo>^</mo></mover><mo>=</mo><msup><mn>90</mn><mn>0</mn></msup></mstyle></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>F</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi></mrow><mo>^</mo></mover><mo>+</mo><mover accent="true"><mrow><mi>E</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>B</mi></mrow><mo>^</mo></mover><mo>=</mo><mover accent="true"><mrow><mi>x</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>y</mi></mrow><mo>^</mo></mover><mo>=</mo><msup><mn>90</mn><mn>0</mn></msup></mstyle></math><br />Nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover><mo>=</mo><mover accent="true"><mrow><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>F</mi></mrow><mo>^</mo></mover></mstyle></math> (cùng phụ với <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover></mstyle></math>)<br />Xét <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">△</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mstyle></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">△</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>F</mi></mstyle></math> có:<br />+) <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover><mo>=</mo><mover accent="true"><mrow><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>F</mi></mrow><mo>^</mo></mover></mstyle></math> (chứng minh trên)<br />+) OA=OB (O là tâm đối xứng của hình vuông)<br />+) <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>E</mi></mrow><mo>^</mo></mover><mo>=</mo><mover accent="true"><mrow><mi>O</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow><mo>^</mo></mover><mo>=</mo><msup><mn>45</mn><mn>0</mn></msup></mstyle></math> (tính chất hình vuông)<br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mo>⇒</mo><mi mathvariant="normal">Δ</mi><mi>A</mi><mi>O</mi><mi>E</mi><mo>=</mo><mi mathvariant="normal">Δ</mi><mi>B</mi><mi>O</mi><mi>F</mi><mo>(</mo><mi>g</mi><mo>,</mo><mi>c</mi><mo>,</mo><mi>g</mi><mo>)</mo></mstyle></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mo>⇒</mo><msub><mi>S</mi><mrow><mi>A</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>E</mi></mrow></msub><mo>=</mo><msub><mi>S</mi><mrow><mi>B</mi><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>F</mi></mrow></msub></mstyle></math><br />Do đó <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow></msub><mo>=</mo><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⁢</mo><mi>B</mi></mrow></msub><mo>+</mo><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow></msub><mo>=</mo><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⁢</mo><mi>B</mi></mrow></msub><mo>+</mo><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>E</mi></mrow></msub><mo>=</mo><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow></msub></mstyle></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow></msub><mo>=</mo><mfrac><mn>1</mn><mn>2</mn></mfrac><mo>⁢</mo><mi>O</mi><mo>⁢</mo><mi>A</mi><mo>⋅</mo><mi>O</mi><mo>⁢</mo><mi>B</mi><mo>=</mo><mfrac><mn>1</mn><mn>2</mn></mfrac><mo>⋅</mo><mfrac><mn>1</mn><mn>2</mn></mfrac><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>C</mi><mo>⋅</mo><mfrac><mn>1</mn><mn>2</mn></mfrac><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>D</mi></mstyle></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mo>=</mo><mfrac><mn>1</mn><mn>4</mn></mfrac><mo>⋅</mo><mrow><mo>(</mo><mfrac><mn>1</mn><mn>2</mn></mfrac><mi>A</mi><mi>C</mi><mo>.</mo><mi>B</mi><mi>D</mi><mo>)</mo></mrow><mo>=</mo><mfrac><mn>1</mn><mn>4</mn></mfrac><msub><mi>S</mi><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi><mo>⁢</mo><mi>D</mi></mrow></msub></mstyle></math><br />Vậy <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msub><mi>S</mi><mrow><mi>O</mi><mo>⁢</mo><mi>E</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>F</mi></mrow></msub><mo>=</mo><mfrac><mn>1</mn><mn>4</mn></mfrac><mo>⁢</mo><msub><mi>S</mi><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi><mo>⁢</mo><mi>D</mi></mrow></msub><mo>=</mo><mfrac><mn>1</mn><mn>4</mn></mfrac><mo>⁢</mo><msup><mi>a</mi><mn>2</mn></msup></mstyle></math></p>
<p> </p>
Xem lời giải bài tập khác cùng bài
Hướng dẫn giải Bài 41 (Trang 132 SGK Toán Hình học 8, Tập 1)
Xem lời giải
Hướng dẫn giải Bài 42 (Trang 132 SGK Toán Hình học 8, Tập 1)
Xem lời giải
Hướng dẫn giải Bài 44 (Trang 133 SGK Toán Hình học 8, Tập 1)
Xem lời giải
Hướng dẫn giải Bài 45 (Trang 133 SGK Toán Hình học 8, Tập 1)
Xem lời giải
Hướng dẫn giải Bài 46 (Trang 133 SGK Toán Hình học 8, Tập 1)
Xem lời giải
Hướng dẫn giải Bài 47 (Trang 133 SGK Toán Hình học 8, Tập 1)
Xem lời giải