Đề bài

Cho một hình chữ nhật. Vẽ tứ giác có các đỉnh là trung điểm các cạnh của hình chữ nhật . Vì sao tứ giác này là một hình thoi? So sánh diện tích hình thoi và diện tích hình chữ nhật, từ đó suy ra cách tính diện tích hình thoi.

Lời giải chi tiết

Vẽ hình chữ nhật $ABCD$. $M,N,P,Q.$ lần lượt là trung điểm các cạnh AD,AB,BC,CD

Vẽ tứ giác $MNPQ$

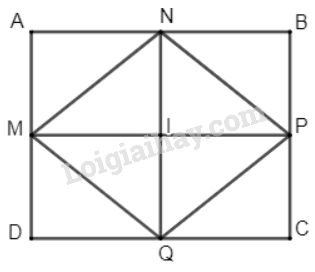
Ta có:

$M$ là đường trung bình của tam giác $ABD$ nên

$MN=\frac{1}{2}BD$ (tính chất)
PQ là đường trung bình của tam giác CBD nên $PQ=\frac{1}{2}BD$ (tính chất)
NP là đường trung bình của tam giác ABC nên $NP=\frac{1}{2}AC$ (tính chất)
MQ là đường trung bình của tam giác ADC nên $MQ=\frac{1}{2}AC$ (tính chất)
Mà $\mathrm{ABCD}$ là hình chữ nhật nên AC=BD (tính chất hình chữ' nhật) nên suy ra MN=PQ=NP=MQ
$\Rightarrow MNPQ$ là hình thoi (dấu hiệu nhận biết hình thoi)
Ta có: $\mathrm{\Delta }AMN=\mathrm{\Delta }INM,\mathrm{\Delta }BPN=\mathrm{\Delta }NIP,$
$\mathrm{\Delta }PCQ=\mathrm{\Delta }IQP,\mathrm{\Delta }DMQ=\mathrm{\Delta }IQM$
$\Rightarrow S_{AMN}=S_{INM},S_{BPN}=S_{NIP}$
$S_{PCQ}=S_{IQP},S_{DMQ}=S_{IQM}$
Ta có:
$S_{MNPQ}=S_{MNI}+S_{NIP}+S_{IQP}+S_{MQI}$
$=S_{AMN}+S_{BNP}+S_{PCQ}+S_{MQD}$
$=\frac{1}{2}{S}_{ABC\mathrm{D}}=\frac{1}{2}\cdot AB.AD$
$=\frac{1}{2}\cdot MP\cdot NQ$
Vậy $MNPQ=\frac{1}{2}MP.NQ$.

Do đó diện tích hình thoi bằng nửa tích hai đường chéo.