Bài 2. Hai đường thẳng vuông góc
Hướng dẫn giải Hoạt động 1 (Trang 93 SGK Toán Hình học 11)
<p><strong class="content_question">Đề bài</strong></p>
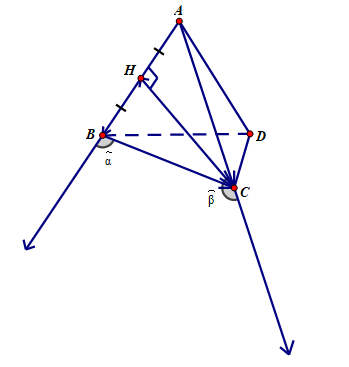
<p>Cho tứ diện đều <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi><mi>D</mi></math> có <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>H</mi></math> là trung điểm của cạnh <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi></math>. Hãy tính góc giữa các cặp vecto sau đây:</p>
<p>a) <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>A</mi><mi>B</mi></mrow><mo>→</mo></mover></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>B</mi><mi>C</mi></mrow><mo>→</mo></mover></math></p>
<p>b) <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>C</mi><mi>H</mi></mrow><mo>→</mo></mover></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>A</mi><mi>C</mi></mrow><mo>→</mo></mover></math></p>
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p><img src="https://img.loigiaihay.com/picture/2018/0917/cau-1-trang-93.PNG" alt="" width="300" height="315" /></p>
<p>Tứ diện <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi><mi>D</mi></math> đều có các mặt là tam giác đều.</p>
<p>a) Góc giữa <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>A</mi><mi>B</mi></mrow><mo>→</mo></mover></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>B</mi><mi>C</mi></mrow><mo>→</mo></mover></math> là góc <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>α</mi></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>α</mi><mo>=</mo><mn>180</mn><mo>°</mo><mo>-</mo><mn>60</mn><mo>°</mo><mo>=</mo><mn>120</mn><mo>°</mo></math></p>
<p>b) Góc giữa <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>C</mi><mi>H</mi></mrow><mo>→</mo></mover></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>A</mi><mi>C</mi></mrow><mo>→</mo></mover></math> là góc <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>β</mi></math></p>
<p> <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>H</mi></math> là trung điểm cạnh <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi></math> của tam giác đều <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi></math> nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>C</mi><mi>H</mi></math> vừa là trung tuyến vừa là đường cao nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>C</mi><mi>H</mi><mo>⊥</mo><mi>A</mi><mi>B</mi></math></p>
<p>Xét tam giác vuông <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>C</mi><mi>H</mi></math> tại <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>H</mi></math> có </p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><mover><mrow><mi>A</mi><mi>C</mi><mi>H</mi></mrow><mo>^</mo></mover><mo>+</mo><mover><mrow><mi>C</mi><mi>A</mi><mi>H</mi></mrow><mo>^</mo></mover><mo>=</mo><mn>90</mn><mo>°</mo><mo>⇒</mo><mover><mrow><mi>A</mi><mi>C</mi><mi>H</mi></mrow><mo>^</mo></mover><mo>=</mo><mn>90</mn><mo>°</mo><mo>-</mo><mn>60</mn><mo>°</mo><mo>=</mo><mn>30</mn><mo>°</mo></math></p>
<p>Nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>β</mi><mo>=</mo><mn>180</mn><mo>°</mo><mo>-</mo><mn>30</mn><mo>°</mo><mo>=</mo><mn>150</mn><mo>°</mo></math></p>
Xem lời giải bài tập khác cùng bài
Lý thuyết hai đường thẳng vuông góc
Xem lời giải
Hướng dẫn giải Hoạt động 2 (Trang 94 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Hoạt động 3 (Trang 95 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Hoạt động 4 (Trang 97 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Hoạt động 5 (Trang 97 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 1 (Trang 97 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 2 (Trang 97 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 3 (Trang 97 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 4 (Trang 98 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 5 (Trang 98 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 6 (Trang 98 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 7 (Trang 98 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 8 (Trang 98 SGK Toán Hình học 11)
Xem lời giải