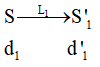5. Một thấu kính mỏng phẳng - lõi L₁ có tiêu cự f₁ = 60 cm được ghép sát đồng trục với một thấu kính mỏng phẳng - lồi khác L₂ có tiêu cự f₂ = 30 cm. Mặt phẳng của hai thấu kính sát nhau.

Thấu kính L₁ có đường kính rìa gấp đôi đường kính rìa của thấu kính L₂. Một điểm sáng S nằm trên trục chính của hệ, trước L₁.  

a) Chứng tỏ có hai ảnh của S được tạo bởi hệ.

b) Tìm điều kiện về vị trí của S để hai ảnh đều thật và hai ảnh đều ảo.

Giải

a)

Khi chùm tia sáng từ S tới các điểm tới từ miền vành ngoài của thấu kính L₂ trở ra thì chỉ đi qua thấu kính L₁ và chùm tia ló sẽ tạo ảnh S₁

Còn chùm tia sáng từ S tới các điểm tới trong trong khoảng từ tâm thấu kính tới miền vành của thấu kính L₂ thì đi qua cả hai thấu kính L₁ và L₂ và chùm tia ló sẽ tạo ảnh S₂.

Sơ đồ tạo ảnh qua thấu kính L₁

Sơ đồ tạo ảnh của hệ hai thấu kính đồng trục:

Trong đó: 

f₂ = 30cm

Trong đó: 

D₁₂ = D₁ +D₂

$\Rightarrow \frac{}{}$

Vì f₂ ≠ f₁₂ ⇒ d₁’ ≠ d₂’

⇒ Hai hình ảnh S₁ và S₂ không trùng nhau

b) Vì  nên:

* Điều kiện để hai ảnh S₁, S₂ đều thật là: d₁ và d₂ > f_max=f1=60cm

* Điều kiện để hai ảnh S₁, S₂ đều ảo là∶d₁ và d₂ < f_min=f₁₂=20cm