Bài 5. Trường hợp đồng dạng thứ nhất
Hướng dẫn giải Bài 29 (Trang 74 SGK Toán Hình học 8, Tập 2)
<p><strong class="content_question">Đề bài</strong></p>
<p>Cho tam giác <span id="MathJax-Element-1-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi></math>"><span id="MJXc-Node-1" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-2" class="mjx-mrow"><span id="MJXc-Node-3" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-4" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">BC</span></span></span></span></span> và <span id="MathJax-Element-2-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mi>A</mi><mo>&#x2032;</mo></msup><msup><mi>B</mi><mo>&#x2032;</mo></msup><msup><mi>C</mi><mo>&#x2032;</mo></msup></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mi>A</mi><mo>′</mo></msup><msup><mi>B</mi><mo>′</mo></msup><msup><mi>C</mi><mo>′</mo></msup></math></span></span> có kích thước như trong hình 35.</p>
<p><img src="https://img.loigiaihay.com/picture/2018/0718/b29-trang-74-sgk-toan-8-t2-c2.jpg" /></p>
<p>a) Tam giác <span id="MathJax-Element-3-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mi>B</mi><mi>C</mi></math></span></span> và <span id="MathJax-Element-4-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mi>A</mi><mo>&#x2032;</mo></msup><msup><mi>B</mi><mo>&#x2032;</mo></msup><msup><mi>C</mi><mo>&#x2032;</mo></msup></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><msup><mi>A</mi><mo>′</mo></msup><msup><mi>B</mi><mo>′</mo></msup><msup><mi>C</mi><mo>′</mo></msup></ma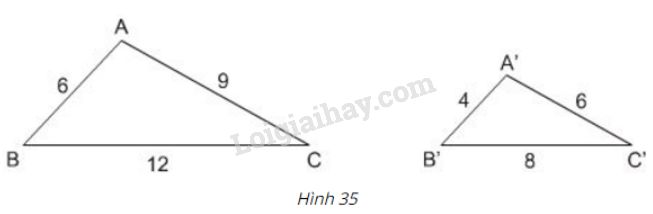th></span></span> có đồng dạng với nhau không? Vì sao?</p>
<p>b) Tính tỉ số chu vi của hai tam giác đó.</p>
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p>Ta có:<br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>⁢</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mfrac><mn>6</mn><mn>4</mn></mfrac><mo>=</mo><mfrac><mn>3</mn><mn>2</mn></mfrac><mo>;</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mfrac><mn>9</mn><mn>6</mn></mfrac><mo>=</mo><mpadded><mfrac><mn>3</mn><mn>2</mn></mfrac></mpadded><mo>;</mo><mo> </mo><mfrac><mrow><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mfrac><mn>12</mn><mn>8</mn></mfrac><mo>=</mo><mpadded><mfrac><mn>3</mn><mn>2</mn></mfrac></mpadded><mspace linebreak="newline"/><mo>⇒</mo><mfrac><mstyle displaystyle="true"><mi>A</mi><mo>⁢</mo><mi>B</mi></mstyle><mstyle displaystyle="true"><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup></mstyle></mfrac><mo>=</mo><mfrac><mstyle displaystyle="true"><mi>A</mi><mo>⁢</mo><mi>C</mi></mstyle><mstyle displaystyle="true"><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mstyle></mfrac><mo>=</mo><mfrac><mstyle displaystyle="true"><mi>B</mi><mo>⁢</mo><mi>C</mi></mstyle><mstyle displaystyle="true"><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mstyle></mfrac><mo>=</mo><mpadded><mfrac><mstyle displaystyle="true"><mn>3</mn></mstyle><mstyle displaystyle="true"><mn>2</mn></mstyle></mfrac></mpadded></math></p>
<p><span id="MathJax-Element-6-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: 400; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: 0px; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; color: #000000; font-family: OpenSans, Tahoma, Helvetica, sans-serif; font-variant-ligatures: normal; font-variant-caps: normal; orphans: 2; widows: 2; -webkit-text-stroke-width: 0px; text-decoration-thickness: initial; text-decoration-style: initial; text-decoration-color: initial; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo stretchy="false">&#x21D2;</mo><mi mathvariant="normal">&#x0394;</mi><mi>A</mi><mi>B</mi><mi>C</mi><mtext>&#xA0;&#x111;&#x1ED3;ng d&#x1EA1;ng&#xA0;</mtext><mi mathvariant="normal">&#x0394;</mi><msup><mi>A</mi><mo>&#x2032;</mo></msup><msup><mi>B</mi><mo>&#x2032;</mo></msup><msup><mi>C</mi><mo>&#x2032;</mo></msup></math>"><span id="MJXc-Node-186" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-187" class="mjx-mrow"><span id="MJXc-Node-188" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">⇒</span></span><span id="MJXc-Node-189" class="mjx-mi MJXc-space3"><span class="mjx-char MJXc-TeX-main-R">Δ</span></span><span id="MJXc-Node-190" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span><span id="MJXc-Node-191" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span><span id="MJXc-Node-192" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span><span id="MJXc-Node-193" class="mjx-mtext"><span class="mjx-char"><span class="mjx-charbox MJXc-TeX-main-R"> </span><span class="mjx-charbox MJXc-TeX-unknown-R">đ</span><span class="mjx-charbox MJXc-TeX-unknown-R">ồ</span><span class="mjx-charbox MJXc-TeX-main-R">ng d</span><span class="mjx-charbox MJXc-TeX-unknown-R">ạ</span><span class="mjx-charbox MJXc-TeX-main-R">ng </span></span></span><span id="MJXc-Node-194" class="mjx-mi"><span class="mjx-char MJXc-TeX-main-R">Δ</span></span><span id="MJXc-Node-195" class="mjx-msup"><span class="mjx-base"><span id="MJXc-Node-196" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">A</span></span></span><span class="mjx-sup"><span id="MJXc-Node-197" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">′</span></span></span></span><span id="MJXc-Node-198" class="mjx-msup"><span class="mjx-base"><span id="MJXc-Node-199" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">B</span></span></span><span class="mjx-sup"><span id="MJXc-Node-200" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">′</span></span></span></span><span id="MJXc-Node-201" class="mjx-msup"><span class="mjx-base"><span id="MJXc-Node-202" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">C</span></span></span><span class="mjx-sup"><span id="MJXc-Node-203" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">′</span></span></span></span></span></span></span> <span id="MathJax-Element-7-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: 400; font-size: 16.94px; letter-spacing: normal; overflow-wrap: normal; word-spacing: 0px; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; color: #000000; font-family: OpenSans, Tahoma, Helvetica, sans-serif; font-variant-ligatures: normal; font-variant-caps: normal; orphans: 2; widows: 2; -webkit-text-stroke-width: 0px; text-decoration-thickness: initial; text-decoration-style: initial; text-decoration-color: initial; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mrow><mo>(</mo><mrow><mi>c</mi><mo>&#x2212;</mo><mi>c</mi><mo>&#x2212;</mo><mi>c</mi></mrow><mo>)</mo></mrow></math>"><span id="MJXc-Node-204" class="mjx-math" aria-hidden="true"><span id="MJXc-Node-205" class="mjx-mrow"><span id="MJXc-Node-206" class="mjx-mrow"><span id="MJXc-Node-207" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">(</span></span><span id="MJXc-Node-208" class="mjx-mrow"><span id="MJXc-Node-209" class="mjx-mi"><span class="mjx-char MJXc-TeX-math-I">c</span></span><span id="MJXc-Node-210" class="mjx-mo MJXc-space2"><span class="mjx-char MJXc-TeX-main-R">−</span></span><span id="MJXc-Node-211" class="mjx-mi MJXc-space2"><span class="mjx-char MJXc-TeX-math-I">c</span></span><span id="MJXc-Node-212" class="mjx-mo MJXc-space2"><span class="mjx-char MJXc-TeX-main-R">−</span></span><span id="MJXc-Node-213" class="mjx-mi MJXc-space2"><span class="mjx-char MJXc-TeX-math-I">c</span></span></span><span id="MJXc-Node-214" class="mjx-mo"><span class="mjx-char MJXc-TeX-main-R">)</span></span></span></span></span></span><br />b) Áp dụng tính chất của dãy tỉ số bằng nhau ta có:<br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></mfrac><mo>=</mo><mpadded><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>+</mo><mi>A</mi><mo>⁢</mo><mi>C</mi><mo>+</mo><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup><mo>+</mo><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup><mo>+</mo><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></mfrac></mpadded><mo>=</mo><mfrac><msub><mi>C</mi><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow></msub><msub><mi>C</mi><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></msub></mfrac><mo>=</mo><mfrac><mn>3</mn><mn>2</mn></mfrac></math><br />(với <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msub><mi>C</mi><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi></mrow></msub></mstyle></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msub><mi>C</mi><mrow><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mrow></msub></mstyle></math> lần lượt là chu vi của hai tam giác <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi><mo>,</mo><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mstyle></math>)<br />Vậy tỉ số chu vi của tam giác ABC và chu vi của tam giác <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><msup><mi>A</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>B</mi><mo>'</mo></msup><mo>⁢</mo><msup><mi>C</mi><mo>'</mo></msup></mstyle></math> là <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mn>3</mn><mn>2</mn></mfrac></mstyle></math></p>
<p> </p>
<p> </p>
Xem lời giải bài tập khác cùng bài