Bài 6. Trường hợp đồng dạng thứ hai
Hướng dẫn giải Bài 34 (Trang 77 SGK Toán Hình học 8, Tập 2)
<p>Đề bài<br />Dựng tam giác ABC, biết <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mi>A</mi><mo>^</mo></mover><mo>=</mo><msup><mn>60</mn><mo>∘</mo></msup></mstyle></math> và, tỉ số <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mn>4</mn><mn>5</mn></mfrac></mstyle></math> và đường cao <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>A</mi><mo>⁢</mo><mi>H</mi><mo>=</mo><mpadded><mn>6</mn></mpadded><mo>⁢</mo><mi>cm</mi><mo>.</mo></math></p>
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p><strong class="content_detail"><img src="https://img.loigiaihay.com/picture/2019/0314/h13-bai-34-trang-77-sgk-toan-8-t2.jpg" /></strong></p>
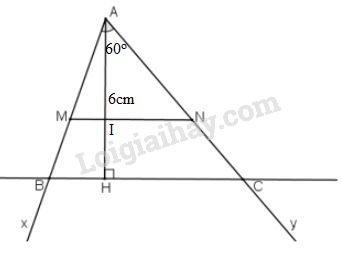
<p><span class="content_detail">Cách dự̛ng:<br />- Dựng <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>x</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>y</mi></mrow><mo>^</mo></mover><mo>=</mo><msup><mn>60</mn><mo>∘</mo></msup></mstyle></math><br />- Trên hai cạnh Ax, Ay của góc <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mover accent="true"><mrow><mi>x</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>y</mi></mrow><mo>^</mo></mover></mstyle></math> lần lượt dựng <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi>A</mi><mo>⁢</mo><mi>M</mi><mo>=</mo><mpadded><mn>4</mn></mpadded><mo>⁢</mo><mi>cm</mi><mo>,</mo><mi>A</mi><mo>⁢</mo><mi>N</mi><mo>=</mo><mpadded><mn>5</mn></mpadded><mo>⁢</mo><mi>cm</mi></mstyle></math>. Kẻ đường cao AI của <math xmlns="http://www.w3.org/1998/Math/MathML"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>M</mi><mo>⁢</mo><mi>N</mi><mo>.</mo></math><br />- Trên tia AI lấy điểm H sao cho <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi>A</mi><mo>⁢</mo><mi>H</mi><mo>=</mo><mpadded><mn>6</mn></mpadded><mo>⁢</mo><mi>cm</mi></mstyle></math>, qua H vẽ đường song song với MN cắt Ax, Ay lần Iượt tại B và C<br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>⇒</mo><mi mathvariant="normal">△</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi></math> thỏa mãn điều kiện bài toán.<br />Chứng minh:<br />Áp dụng định lí: Một đường thẳng cắt hai cạnh của tam giác và song song với cạnh còn lại tạo thành một tam giác đồng dạng với tam giác đã cho ta có:<br />MN//BC (theo cách dựng)<br />Suy ra <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi mathvariant="normal">Δ</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>M</mi><mo>⁢</mo><mi>N</mi></mstyle></math> đồng dạng <math xmlns="http://www.w3.org/1998/Math/MathML"><mi mathvariant="normal">△</mi><mo>⁢</mo><mi>A</mi><mo>⁢</mo><mi>B</mi><mo>⁢</mo><mi>C</mi><mo>.</mo></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mo>⇒</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>M</mi></mrow><mrow><mi>A</mi><mo>⁢</mo><mi>N</mi></mrow></mfrac><mo>=</mo><mfrac><mrow><mi>A</mi><mo>⁢</mo><mi>B</mi></mrow><mrow><mi>A</mi><mo>⁢</mo><mi>C</mi></mrow></mfrac><mo>=</mo><mfrac><mn>4</mn><mn>5</mn></mfrac></mstyle></math> (tính chất hai tam giác đồng dạng)<br />Vì <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi>A</mi><mo>⁢</mo><mi>H</mi><mo>⟂</mo><mi>M</mi><mo>⁢</mo><mi>N</mi></mstyle></math>, mà MN//BC nên <math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mi>A</mi><mo>⁢</mo><mi>H</mi><mo>⟂</mo><mi>B</mi><mo>⁢</mo><mi>C</mi><mo>,</mo><mi>A</mi><mo>⁢</mo><mi>H</mi><mo>=</mo><mpadded><mn>6</mn></mpadded><mo>⁢</mo><mi>cm</mi></mstyle></math><br /><math xmlns="http://www.w3.org/1998/Math/MathML"><mstyle displaystyle="false"><mo>⇒</mo><mi>A</mi><mo>⁢</mo><mi>H</mi></mstyle></math> là đường cao của tam giác ABC.<br /></span><span class="content_detail">Vậy tam giác ABC thỏa mãn yêu cầu bài toán.</span><strong class="content_detail"><br /></strong></p>
Xem lời giải bài tập khác cùng bài