Bài 4. Hai mặt phẳng song song
Hướng dẫn giải Hoạt động 2 (Trang 65 SGK Toán Hình học 11)
<p><strong class="content_question">Đề bài</strong></p>
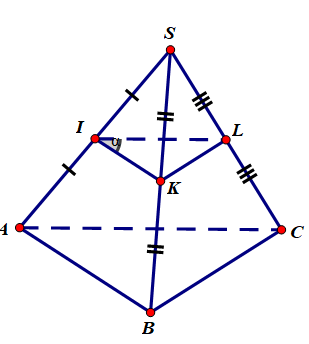
<p>Cho tứ diện <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mo> </mo><mi>A</mi><mi>B</mi><mi>C</mi></math>. Hãy dựng mặt phẳng <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math> qua trung điểm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi></math> của đoạn <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>A</mi></math>và song song với mặt phẳng <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced></math>.</p>
<p><img src="https://img.loigiaihay.com/picture/2018/0915/bai-4-trang-65.PNG" alt="" width="201" height="224" /></p>
<p class="content_method_header"><strong class="content_method">Phương pháp giải </strong></p>
<div class="content_method_content">
<p><em><strong>Cách 1</strong></em>: Xác định mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math>:</p>
<p>Gọi các giao điểm của <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math> với các cạnh <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi></math><span id="MathJax-Element-8-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi><mo>.</mo></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>.</mo></math></span></span> Chỉ ra đặc điểm và xác định vị trí của các giao điểm ấy.</p>
<p><em><strong>Cách 2</strong></em>: Lấy K, L là trung điểm của SB, SC. Chứng minh: <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced><mo>≡</mo><mfenced><mrow><mi>I</mi><mi>K</mi><mi>L</mi></mrow></mfenced></math></p>
</div>
<p><strong class="content_detail">Lời giải chi tiết</strong></p>
<p><strong>Cách 1:</strong></p>
<p>Gọi <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>K</mi><mo>,</mo><mi>L</mi></math> lần lượt là giao của mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math> với các cạnh <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi></math> <span id="MathJax-Element-12-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi><mo>.</mo></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>.</mo></math></span></span></p>
<p>Ta có: <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mspace linebreak="newline"></mspace><mo>⇒</mo><mfenced open="{" close=""><mtable columnalign="left"><mtr><mtd><mi>I</mi><mi>K</mi><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mo>⊃</mo><mi>A</mi><mi>B</mi></mtd></mtr><mtr><mtd><mi>I</mi><mi>L</mi><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mo>⊃</mo><mi>A</mi><mi>C</mi></mtd></mtr></mtable></mfenced><mspace linebreak="newline"></mspace><mo>⇒</mo><mfenced open="{" close=""><mtable columnalign="left"><mtr><mtd><mi>I</mi><mi>K</mi><mo>∥</mo><mi>A</mi><mi>B</mi></mtd></mtr><mtr><mtd><mi>I</mi><mi>L</mi><mo>∥</mo><mi>A</mi><mi>C</mi></mtd></mtr></mtable></mfenced></math></p>
<p>Mà <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi></math> là trung điểm của <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>A</mi></math><span id="MathJax-Element-16-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>A</mi><mo>.</mo></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>.</mo></math></span></span></p>
<p><span id="MathJax-Element-17-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo stretchy="false">&#x21D2;</mo><mrow><mo>{</mo><mtable columnalign="left" rowspacing="4pt" columnspacing="1em"><mtr><mtd><mi>K</mi><mtext>l&#xE0; trung &#x111;i&#x1EC3;m c&#x1EA1;nh SB</mtext></mtd></mtr><mtr><mtd><mi>I</mi><mspace width="thickmathspace" /><mtext>l&#xE0; trung &#x111;i&#x1EC3;m c&#x1EA1;nh SC</mtext></mtd></mtr></mtable><mo fence="true" stretchy="true" symmetric="true"></mo></mrow></math>"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>⇒</mo><mfenced open="{" close=""><mtable columnalign="left"><mtr><mtd><mi>K</mi><mo> </mo><mi>l</mi><mi>à</mi><mo> </mo><mi>t</mi><mi>r</mi><mi>u</mi><mi>n</mi><mi>g</mi><mo> </mo><mi>đ</mi><mi>i</mi><mi>ể</mi><mi>m</mi><mo> </mo><mi>c</mi><mi>ạ</mi><mi>n</mi><mi>h</mi><mo> </mo><mi>S</mi><mi>B</mi></mtd></mtr><mtr><mtd><mi>I</mi><mo> </mo><mi>l</mi><mi>à</mi><mo> </mo><mi>t</mi><mi>r</mi><mi>u</mi><mi>n</mi><mi>g</mi><mo> </mo><mi>đ</mi><mi>i</mi><mi>ể</mi><mi>m</mi><mo> </mo><mi>c</mi><mi>ạ</mi><mi>n</mi><mi>h</mi><mo> </mo><mi>S</mi><mi>C</mi></mtd></mtr></mtable></mfenced></math></span></p>
<p>Vậy mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math> chính là mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>I</mi><mi>K</mi><mi>L</mi></mrow></mfenced></math><span id="MathJax-Element-19-Frame" class="mjx-chtml MathJax_CHTML" style="margin: 0px; padding: 1px 0px; display: inline-block; line-height: 0; text-indent: 0px; text-align: left; text-transform: none; font-style: normal; font-weight: normal; font-size: 21.78px; letter-spacing: normal; overflow-wrap: normal; word-spacing: normal; white-space: nowrap; float: none; direction: ltr; max-width: none; max-height: none; min-width: 0px; min-height: 0px; border: 0px; position: relative;" tabindex="0" role="presentation" data-mathml="<math xmlns="http://www.w3.org/1998/Math/MathML"><mo stretchy="false">(</mo><mi>I</mi><mi>K</mi><mi>L</mi><mo stretchy="false">)</mo><mo>.</mo></math>"><span class="MJX_Assistive_MathML" role="presentation"><math xmlns="http://www.w3.org/1998/Math/MathML"><mo>.</mo></math></span></span></p>
<p><strong>Cách 2:</strong></p>
<p>Mặt phẳng <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced></math> là mặt phẳng đi qua 3 trung điểm <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mo>,</mo><mi>K</mi><mo>,</mo><mi>L</mi></math> của <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>A</mi><mo>,</mo><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi></math></p>
<p>Thật vậy, gọi <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>K</mi><mo>,</mo><mi>L</mi></math> lần lượt là trung điểm của <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mo>,</mo><mi>S</mi><mi>C</mi></math></p>
<p>Suy ra <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mi>K</mi><mo>,</mo><mi>K</mi><mi>L</mi></math> lần lượt là đường trung bình trong tam giác <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>A</mi><mi>B</mi></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>S</mi><mi>B</mi><mi>C</mi></math></p>
<p><math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mi>K</mi><mo>∥</mo><mi>A</mi><mi>B</mi><mo>∈</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mo>⇒</mo><mi>I</mi><mi>K</mi><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mspace linebreak="newline"></mspace><mi>K</mi><mi>L</mi><mo>∥</mo><mi>B</mi><mi>C</mi><mo>∈</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced><mo>⇒</mo><mi>K</mi><mi>L</mi><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced></math></p>
<p> <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mi>K</mi></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>K</mi><mi>L</mi></math> cắt nhau và cùng song song với mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced></math></p>
<p>⇒ Mặt phẳng chứa <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>I</mi><mi>K</mi></math> và <math xmlns="http://www.w3.org/1998/Math/MathML"><mi>K</mi><mi>L</mi></math> song song với mp <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced></math></p>
<p>Hay <math xmlns="http://www.w3.org/1998/Math/MathML"><mfenced><mi>α</mi></mfenced><mo>∥</mo><mfenced><mrow><mi>A</mi><mi>B</mi><mi>C</mi></mrow></mfenced></math></p>
Xem lời giải bài tập khác cùng bài
Lý thuyết Hai mặt phẳng song song
Xem lời giải
Hướng dẫn giải Hoạt động 1 (Trang 64 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Hoạt động 3 (Trang 68 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 1 (Trang 71 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 2 (Trang 71 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 3 (Trang 71 SGK Toán Hình học 11)
Xem lời giải
Hướng dẫn giải Bài 4 (Trang 71 SGK Toán Hình học 11)
Xem lời giải